This research focuses on the definition of polynomial surrogates for the effective strain energy function (potential) defining the homogenized behavior of nonlinear microstructures. To this aim, the effective potential is approximated in a multivariate polynomial space where basis functions are orthogonal with respect to the d-dimensional uniform probability measure, the latter being associated with the components of the macroscopic deformation gradient. From a stochastic standpoint, this construction corresponds to a polynomial chaos expansion (of the homogenized strain energy function), the coefficients of which are computed by a Gauss-Legendre quadrature rule.
Additionally, this framework allows us to define projections of the effective potential onto given classes of nonlinear potentials, such as Ogden-type functions. Various benchmarks on isotropic and anisotropic microstructures were performed, and it was shown that the surrogate model and the projected potential both yield very accurate approximations - even at large mechanical contrasts. The projection scheme turns out to be particularily interesting from a engineering standpoint, as it allows nonlinear multiscale predictions to be readily and concurrently coupled with structural applications at no additional cost.
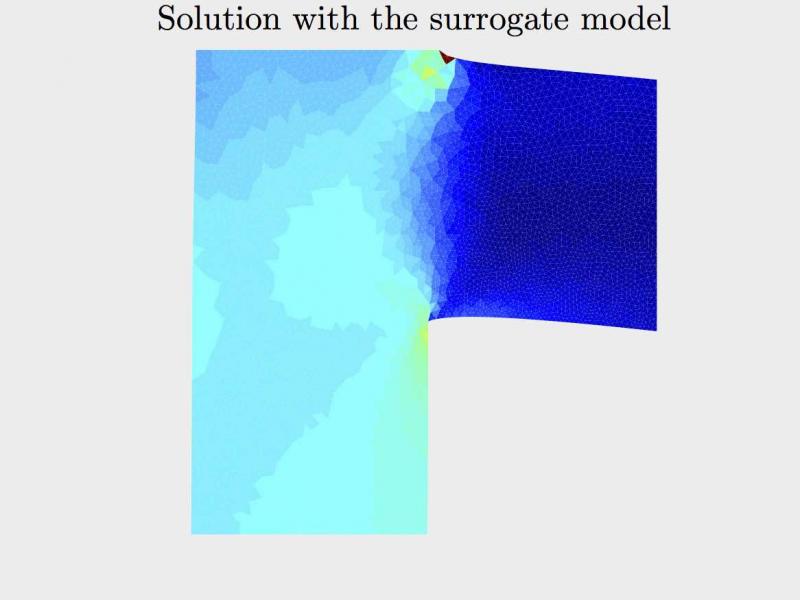
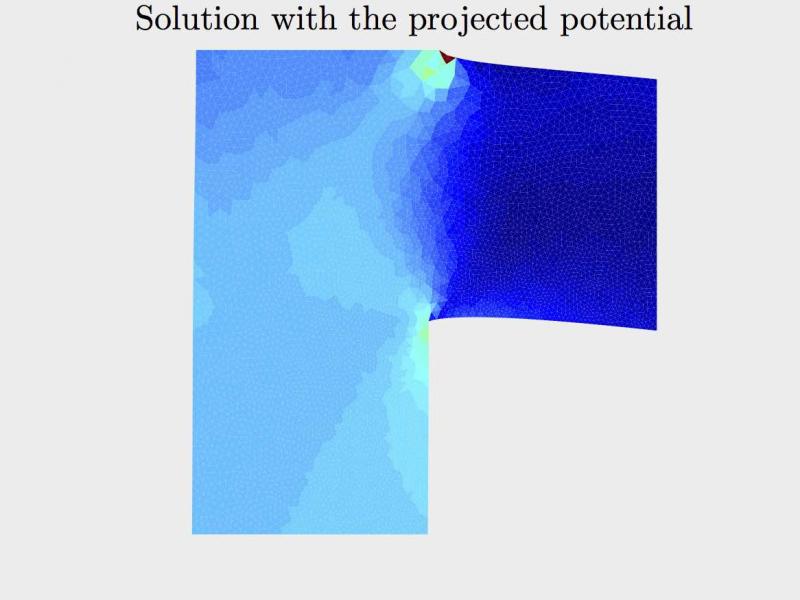
An example of a structural application where the macroscopic and multiscale simulations are coupled (on the fly) is shown below. The reference solution (defined by the so-called FE² solution) and the approximated ones are depicted for the Von Mises stress.
Image

| Image
| Image
|
Comparing the prediction of the Von Mises stress at finite strains: reference solution (left), solution based on the polynomial surrogate (middle) and solution based on the projected potential (right)
For further details, please refer to the following publication:
- B. Staber and J. Guilleminot, Functional approximation and projection of stored energy functions in computational homogenization of hyperelastic materials: a probabilistic perspective, Computer Methods in Applied Mechanics and Engineering, 313, 1-27 (2017)